Question

Please help ASAP! prove the diagonals of the rhombus ABCD bisect each other??

Answer 1

The diagonals of the rhombus ABCD bisect each other

Explanation:

Given: ABCD is a rhombus and AC and BD are diagonals. All sides of the rhombus are equal.

To prove: AC and BD bisects each other.

Let O is the intersection point diagonals.

In triangle ABC, ABD, BCD and ADC are isosceles triangles.

In triangle AOB and COD,

`\angle DBA=\angle BDC`

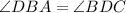
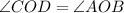`\angle COD=\angle AOB` (Vertically opposite angle)

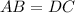`AB=DC` (opposite side of rhombus)

By AAS postulate,

`\triangle COD\cong \triangle AOB`

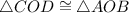
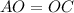`AO=OC` (CPCTC)

O is the midpoint of segment AC. Segment BD bisects segment AC.

In triangle AOD and COB,

`\angle BDA=\angle DBC`

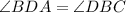
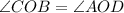`\angle COB=\angle AOD` (Vertically opposite angle)

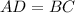`AD=BC` (opposite side of rhombus)

By AAS postulate,

`\triangle AOD\cong \triangle COB`

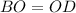`BO=OD` (CPCTC)

Segment BO is congruent to segment OD.

O is the midpoint of segment BD. Segment AC bisects segment BD.

Hence proved.