Question

A square has a side length of 7 centimeters. A circle has a diameter of 7 centimeters. What is true about the areas of the two figures?

Answer 1

Answer: The area of the circle will be eleven-fourteenth of the area of square.

Step-by-step explanation: Given that a square has a side of length 7 cm and a circle has a diameter of 7 cm. We need to find the statement which is true about the areas of these two figures.

The area of the square will be

`S_a=7^2=49~\textup{cm}^2.`

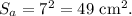
And, the area of the circle will be

`C_a=\pi r^2=\pi * \left((7)/(2)\right)^2=(\pi)/(4)* 49=(22)/(7* 4)* 49\\\\\Rightarrow C_a=(11)/(14)* S_a.`

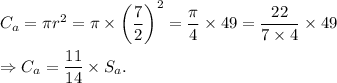
Thus, the area of the circle will be eleven-fourteenth of the areq of the square.

Answer 2

Area of Square having side length equal to 7cm

=(7)²

=49 cm²

because ,Area of square=Side × Side

⇒Area of Circle having diameter 7 cm will be

`\text{radius}=\frac{\text{Diameter}}{2}\\\\\text{Area of circle}=\pi * r^2\\\\=(22)/(7)* ((7)/(2))^2\\\\=(22* 49)/(7* 4)\\\\=(77)/(2)\\\\=38.5 \text{cm}^2`

Area of square is larger than Area of circle by

=49-38.5

=10.5 cm²