Question

A prehistoric site was discovered. The site is a nearly perfect circle, consisting of nine concentric rings that probably held upright wooden posts. Around this timber temple is a wide, encircling ditch enclosing an area with a diameter of 437 ft. Find this enclosed area to the NEAREST THOUSAND square feet. Use 3.14 for π.

Answer 1

Answer: The answer is 1350000 sq. ft.

Step-by-step explanation: Given that a prehistoric site was discovered, which is nearly a perfect circle, consisting of nine concentric rings that probably held upright wooden posts. Around this timber temple is a wide, encircling ditch enclosing an area with a diameter of 437 ft. We need to calculate the enclosed area.

The radius of the encircling ditch is given by

`r=(437)/(2)=218.5~\textup{ft.}`

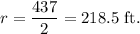
So, the area of this encircling ditch will be

`a=\pi r^2=(22)/(7)* (218.5)^2=150047.07\sim 150000~\textup{sq. ft.}`

Since the site is consisting of 9 concentric rings, so the total enclosed area will be

`A=9a=9* 150000=1350000~\textup{sq. ft.}`

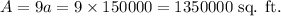
Thus, the required area is 1350000 sq. ft.