Question

A game is played as follows: a die numbered 1 to 6 is rolled. If the number 1 comes up, you get nothing. If the number 2, 3, or 5 comes up, you win $4. If the number 4 or 6 comes up, you lose $6. According to the expected value, this is a fair game. True False

Answer 1

Steps

So before I get to the steps to solving, we should first define what a fair game is. A fair game is a game where there is equal chance of win or loss, so for this to be a fair game, the expected value must be zero.

Now that we defined what a fair game is, let's get to solving! Firstly, we want to get all the possibilities and multiply their outcomes with their chance. In this case the possibilities are {1,2,3,4,5,6}, all have a 1/6 chance, and {1} outcome is 0, {2,3,5} outcome is 4 and {4,6} outcome is -6:

`\textsf{1:}\ 0* (1)/(6)=0\\\\\textsf{2:}\ 4* (1)/(6)=(4)/(6)\\\\\textsf{3:}\ 4* (1)/(6)=(4)/(6)\\\\\textsf{4:}\ -6* (1)/(6)=-(6)/(6)\\\\\textsf{5:}\ 4* (1)/(6)=(4)/(6)\\\\\textsf{6:}\ -6* (1)/(6)=-(6)/(6)`

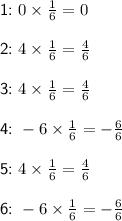
Next, take all the products and add them up:

`(0)/(6)+(4)/(6)+(4)/(6)-(6)/(6)+(4)/(6)-(6)/(6)=(0)/(6)=0`

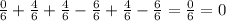
Answer

Since the expected value is zero, it is true that this is a fair game.