Question

What is the vertex of f (x) = 5x^2+20x-16

Answer 1

vertex = (- 2, - 36)

Explanation:

Given a parabola in standard form : y = ax² + bx + c : a ≠ 0

Then the x- coordinate of the vertex is

`x_(vertex)` = -
`(b)/(2a)`

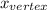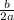
f(x) = 5x² + 20x - 16 is in standard form

with a = 5, b = 20 and c = - 16

`x_(vertex)` = -
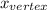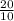`(20)/(10)` = - 2

Substitute x = - 2 into f(x) for corresponding y- coordinate

f(- 2) = 5(- 2)² +20(- 2) - 16 = 20 - 40 - 16 = - 36

vertex = (- 2, - 36)

Answer 2

(-2,-16)

Explanation:

Given is a function

`f(x) =5x^2+20x-16`

To find its vertex

We can use completion of squares method

`f(x) =5x^2+20x-16`

`f(x)=5(x^2+4x)-16\\=5(x^2+4x+4)-20-16\\=5(x+2)^2-16`

This is in std vertex form of a parabola

From the equation we find that the vertex is

(-2,-16)

Hence vertex is (-2,-16)

Verify:

Derivative of f(x) =f'(x)=10x+20

Equate to 0 to have x=-2

f(-2) = 20-40-16=-36

Thus verified