Question

Which of these is NOT a Pythagorean triple? A) 3, 4, 5 B) 6, 8, 10 Eliminate C) 5, 12, 13 D) 8, 40, 41

Answer 1

Option D.
`8,40,41`

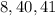
Explanation:

we know that

A "Pythagorean Triple" is a set of positive integers, a, b and c that fits the Pythagorean Theorem

`c^(2)=a^(2)+b^(2)`

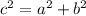
where

c is the greater length side

Verify each case

Case A)
`3,4,5`

`5^(2)=3^(2)+4^(2)`

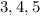
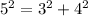
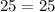`25=25` -----> Is true

therefore

the set of integers is a Pythagorean Triple

Case B)
`6,8,10`

`10^(2)=6^(2)+8^(2)`

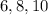
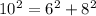
`100=100` -----> Is true

therefore

the set of integers is a Pythagorean Triple

Case C)
`5,12,13`

`13^(2)=5^(2)+12^(2)`

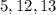
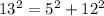
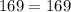`169=169` -----> Is true

therefore

the set of integers is a Pythagorean Triple

Case D)
`8,40,41`

`41^(2)=8^(2)+40^(2)`

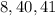
`1,681=1,664` -----> Is not true

therefore

the set of integers is not a Pythagorean Triple