Question

The volume of a cylinder is 108π cm³ and its height is 12 cm. What is the length of the cylinder's radius? Enter your answer in the box.

Answer 1

the radius is 3 cm

Explanation:

The volume of a cylinder is given by

V = pi *r^2 h

We know the volume = 108 *pi and the height = 12

Substituting into the equation

108 * pi = pi * r^2 * 12

Divide each side by pi

108 * pi/pi = pi/pi * r^2 * 12

108 = r^2 * 12

Divide each side by 12

108/12 = r^2*12/12

9 = r^2

Take the square root of each side

sqrt(9) = sqrt(r^2)

3 = r

Answer 2

`\boxed{r=3cm}`

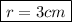
Explanation:

The volume of a cylinder is given by the formula;

`V=\pi \time r^2 h`

It was given that the volume of the cylinder,
`V=108\pi`, and its height,
`h=12cm`

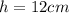
We substitute the given values into the formula to obtain;

`108\pi=\pi* r^2* 12`

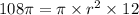
We divide through by
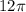`12\pi` to obtain;

`(108\pi)/(12\pi)=r^2`

`\Rightarrow 9=r^2`

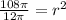
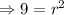
We take the positive square root of both sides to obtain;

`\Rightarrow √(9)=r`

`3=r`

`r=3cm`

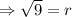
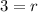
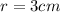
Therefore the length of the cylinder's radius is 3cm.