Question

Can you please help me!

Answer 1

D

Explanation:

When similar triangles are present, you can write ratios which are equal in a proportion. To write the ratios, you must compare corresponding sides either from triangle to triangle or within each triangle.

For instance, focus on triangle BCD and ABC. BCD has the ratio 4/y within its triangle. Since 4 is the short side of BCD and y is the long side of BCD. The same ratio in triangle ABC would be short side to long side. The short side of ABC is y and the long side is 20 so the ratio is y/20.

Putting them together we have
`(4)/(y)=(y)/(20)`

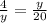
Answer D.