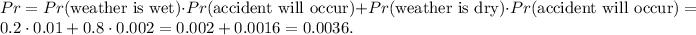Answer:
0.0036
Explanation:
The probabilities that the weather today will be
- wet -
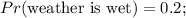
- dry -
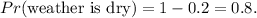
If the weather is wet, then the probabilities that the accident
- will occur -

- will not occur -
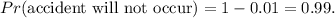
If the weather is dry, then the probabilities that the accident
- will occur -
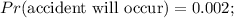
- will not occur -
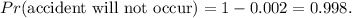
If the weather station announced that there is a 20% chance of the weather being wet, the probability that there will be an accident on highway 17 today is