Question

I need all the parts solved.

Answer 1

(x, y ) = (-4.5, -10)

Explanation:

2x - 3y = 21 ------- eq 1

- 6x + 2y = 7 -------- eq 2

Part 1 : Eliminating the x term

Steps: Multiply eq 1 with 6 and multiply eq 2 with 2 so that value of x becomes equal in both equations

6(2x - 3y) = 21 × 6 ----- 12x - 18y = 126 -----eq 3

2(- 6x + 2y) = 7 × 2---- - 12x + 4y = 14 -----eq 4

The values of x are equal but of opposite sign so we should add the two equaitons to eliminate x

Adding eq3 + eq4:

12x - 18y + ( -12x + 4y ) = 126 + 14

12x - 18y - 12x +4y = 140

-14y = 140

y =
`(140)/(-14)`

y = - 10

Part 2: Eliminating the y term:

Steps: Mulitply eq 1 with 2 and eq 2 with 3 so that the value of y becomes equal in both equations

2(2x - 3y) = 21 × 2 ------- 4x - 6y = 42 ------- eq 5

3( -6x + 2y ) = 7 × 3 ----- -18x + 6y = 21 ----- eq 6

The values of y are equal but of opposite signs so we should add the equation to eliminate y

4x - 6y + (-18x + 6y) = 42 + 21

4x - 6y - 18x + 6y = 63

-14x = 63

x =
`(63)/(-14)`

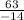
x = - 4.5

Part 3: Choosing the y elimination method to solve the system:

By putting this value of x in eq 1

2x- 3y = 21

2 (-4.5) - 3y = 21

-9 - 3y = 21

-3y = 21 + 9

-3y = 30

y = - 10

Proof:

2x - 3y = 21

2 (- 4.5) - 3(-10) = 21

-9 + 30 = 21

21 = 21