Question

Since BC is parallel to DE, triangles ABC and ADE are similar. What are the lengths of the unknown sides?

A. AC = 14 cm; CE = 8 cm
B. AC = 12 cm; CE = 7 cm
C. AC = 5 cm; CE = 10 cm
D. AC = 10 cm; CE = 5 cm

Answer 1

Option D. AC= 10 cm and CE= 5 cm

Explanation:

From the given figure two triangles ΔABC and ΔADE are similar and two lines BC║DE.

From these similar triangles we know

`(AB)/(AD)=(BC)/(DE)=(AC)/(AE)`

`(8)/(12)=(BC)/(9)=(AC)/(AE)`

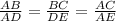
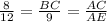
Therefore
`(AC)/(AE)=(8)/(12)=(2)/(3)`

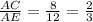
Or AC=
`(2)/(3)AE`

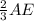
Now from pythagoras theorem

AE = √(AD²+ED²) = √(12²+9²) = √(144+81) =√225 = 15 cm

Since we know side AC =
`(2)/(3)AE`

=
`(2)/(3)* 15`

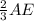
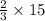
= 10 cm

Therefore AC = 10 cm and AE = 15cm and CE = (15-10) = 5 cm