Question

Solving Quadratic Equations by Factoring

The graph of f(x) = 3x^3 - 5x - 12 is shown


a. Write the equation in intercept form by factoring.


b. What are the x-intercepts of the graph?

What are the zeros of the function?

What are the roots of the function?


c. What are the solutions of 3x^2 - 5x - 12 = 0

Answer 1

The required solutions are a.
`f(x)=(x-3)(3x+4)`, b.
`3\text{ and }-(3)/(4)`, c.
`3\text{ and }-(3)/(4)`.

Explanation:

a.

The given quadratic equation is

`f(x)=3x^2-5x-12`

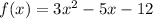
The middle term can be written as (-9x+4x)

`f(x)=3x^2-9x+4x-12`

`f(x)=3x(x-3)+4(x-3)`

`f(x)=(x-3)(3x+4)`

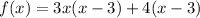
The intercept form of the given equation by factoring. is,

`f(x)=(x-3)(3x+4)`

b.

The x-intercepts,zeros, roots and solutions are same things.

`0=(x-3)(3x+4)`

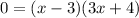
Equate each factor equal to 0.

`x=3,-(3)/(4)`

Therefore x-intercepts,zeros, roots and solutions of the given equation are
`3\text{ and }-(3)/(4)`.

c.

The given equation is

`3x^2-5x-12=0`

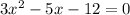
Therefore the solution of the equation are
`3\text{ and }-(3)/(4)`.