Answer:
The product of two numbers changes by 2% when the first number increases by 70%, while the second number decreases by 40%.
Explanation:
Let first number be x and second number be y .
Then product of x and y = xy ......(1)
Given the first number increases by 70%, while the second number decreases by 40% this means,
x is increased by 70% that is
increased value of x = x + 0.7(x) = 1.7 x
Similarly y is decreases by 40% that is
decreased value of y = y - 0.4(y) = 0.6y
New product will be = (increased value of x )( decreased value of y)
= (1.7x)(0.6y)
=1.02xy
Changed Percentage =
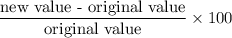
Substitute the values, we get,
Changed Percentage =

Changed Percentage =
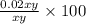
Changed Percentage =
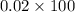
Changed Percentage =
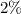
Thus, the product of two numbers changes by 2% when the first number increases by 70%, while the second number decreases by 40%.