3) We have

which has vertical asymptotes (i.e. infinite discontinuities) whenever the denominator is zero. This happens for
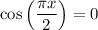

(where
 is any integer)
is any integer)
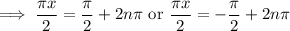
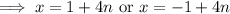
So the graph of
 has vertical asymptotes whenever
has vertical asymptotes whenever
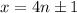 and
and
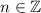 .
.
4) Given
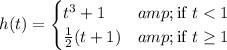
we have the one-sided limits
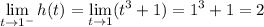
and

The one-sided limits don't match, so the two-sided limit
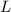 does not exist. In other words, the limit does not exist at
does not exist. In other words, the limit does not exist at
 because the function approaches different values from the left and right side of
because the function approaches different values from the left and right side of
 .
.