Question

Alex is building a rectangle fence around his yard. the total perimeter of the fence is 68 feet and the area of his yard is 240 square feet. Based on this information, what are the dimensions of the fence?

Select one:
A. 4 feet by 10 feet
B. 8 feet by 15 feet
C. 10 feet by 24 feet
D. Cannot be determined from the information given.

Answer 1

Let's call the length of the rectangular fence
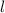`l` and the width of the rectangular fence
`w`.

Based on the information in the problem, we can make two equations, based on the formulas for perimeter and area:

`A = lw`

`P = 2l + 2w`

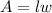
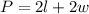
Now, let's substitute in the values we are given in the problem:

`240 = lw`

`68 = 2l + 2w`

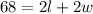
Now, let's solve the system using substitution.

`240 = lw \Rightarrow (240)/(w) = l`

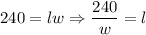
• Solve for
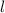  `l` from the first equation

`68 = 2\Bigg( (240)/(w) \Bigg) + 2w`

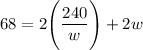
• Substitute this value into the second equation

`68 = (480)/(w) + 2w`

• Simplify

`68w = 480 + 2w^2`

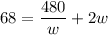
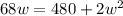
• Multiply both sides of the equation by
  `w`

`2w^2 - 68w + 480 = 0`

• Subtract
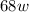  `68w` from both sides of the equation

`(2w - 48)(w - 10) = 0`

• Factor

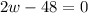`2w - 48 = 0` and
`w - 10 = 0`

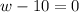
• Use the Zero Product Property and solve both factors

`2w - 48 = 0 \Rightarrow 2w = 48 \Rightarrow w = 24`

`w - 10 = 0 \Rightarrow w = 10`

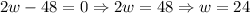
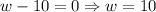
We are given two possible lengths. However, the funny thing is that both produce the same dimensions: 24 ft by 10 ft:

`w = 24 \Rightarrow 240 = l(24) \Rightarrow l = 10`

`w = 10 \Rightarrow 240 = l(10) \Rightarrow l = 24`

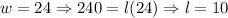
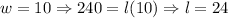
Thus, our answer is Choice C, or 10 feet by 24 feet.