Question

What function equation is represented by the graph?

f(x)=−2x−3

f(x)=−2x−2

f(x)=2x−3

f(x)=2x−2

Answer 1

it's the last answer because the line touches at -2 so the y intercept would be -2 and since the line is going to the up direction it's a positive slope and the last one is a positive so that's why.

Answer 2

`f(x)=2^x-3`

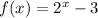
Explanation:

This is an exponential function, in these kind of function the independent variable appears in the exponent and its base is a constant. Its expression is given by:

`f(x)=a^x\\\\a\\eq \pm1`

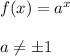
If
`a>0` the exponential function is an increasing function, and if
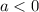`a<0` the exponential function is a decreasing function, on the other hand, exponential functions always pass through the points (0, 1) and (1, a), because:

`f(0)=a^0=1\\f(1)=a^1=a`

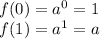
Considering the previous information, you can conclude:

`a>0`

Because from the graph, you can note that it is an increasing function.

Therefore, from the options:

`a=2`

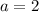
On the other hand the graph pass through the point (0, -2), this means that the graph was translate h units down.

`f(x)=2^x-h`

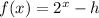
So, let's find h:

`f(0)=-2=2^0 - h\\\\f(0)=-2=1-h`

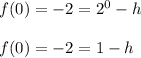
Solving for h:

`h=3`

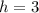
Hence, the equation represented by the graph is:

`f(x)=2^x-3`

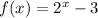
I attached you the graph of this function, so you can corroborate the answer easily.