Question

Rewrite the function in standard form, intercept form, find the vertex, find the y-intercept, and find the x-intercepts.

Please show your work

y=(x-1)^2-9

Answer 1

The function is given as
`y=(x-1)^(2)-9`

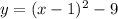
We will simplify the function,

i.e.
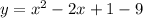`y=x^2-2x+1-9` gives
`y=x^2-2x-8`

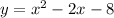
Therefore, the standard form is given by
`f(x)=x^2-2x-8`.

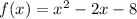As, the given functions have factors (x-4) and (x+2).

Since, the intercept form of a function is its factored form.

Thus, the intercept form is
`f(x)=(x+4)(x-2)`.

Now, it is known that,

X-coordinate of the vertex is
`(-b)/(2a)` i.e.
`(2)/(2* 1)` i.e.
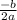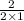`(2)/(2)` i.e. x= 1

So, the value of y at x=1 is,
`y=1^2-2* 1-8` i.e.
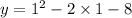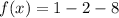`f(x)=1-2-8` i.e. y= -9

Thus, the vertex of the given function is (1,-9).

Since, 'the y-intercept of a function is the point where the function crosses y-axis'.

At x=0, we have,
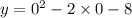`y=0^2-2* 0-8` i.e. y= -8

Therefore, the y-intercept is (0,-8)

And, 'the x-intercept of a function is the point where the function crosses x-axis'.

So, for y=0, we have
`0=x^2-2x-8` i.e.
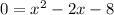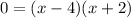`0=(x-4)(x+2)` i.e. x= 4 and x= -2.

Thus, the x-intercept are (4,0) and (-2,0).