Answer:
The velocity of the package relative to the helicopter is 9.9 m/s down.
(B) is correct option
Step-by-step explanation:
Given that,
Velocity of helicopter = 3.5 m/s
Velocity of package = 13.4 m/s
We need to calculate the velocity of the package relative to the helicopter
Just before hitting the ground
Velocity of package relative to helicopter = Velocity of package relative to ground - Velocity of helicopter relative to ground

Put the value into the formula
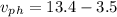
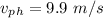
Hence, The velocity of the package relative to the helicopter is 9.9 m/s down.