Question

35 POINTS PLEASE HELP ASAP :)

Find the solution to this system of equations.
y = 6x - 6
2x + 2y = 2

Please give your answer as an ordered pair.

Find the solution to this system of equations.
y = -3x + 5
5x - 4y = -3

Please give your answer as an ordered pair.

Answer 1

Steps

1.

So with this system, I will be using the substitution method. Since we know that y = 6x - 6, substitute y in the second equation for 6x - 6 and solve for x as such:

`2x+2(6x-6)=2\\2x+12x-12=2\\14x-12=2\\14x=14\\x=1`

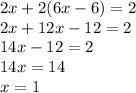
Since we know the value of x is 1, substitute it into either equation to solve for y:

`y=6*1-6\\y=6-6\\y=0\\\\2*1+2y=2\\2+2y=2\\2y=0\\y=0`

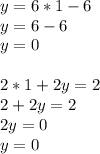
In the form of (x,y), the answer is (1,0).

2.

For this system, I will also be using the substitution method. Since we know that y = -3x + 5, substitute that into the second equation to solve for x as such:

`5x-4(-3x+5)=-3\\5x+12x-20=-3\\17x-20=-3\\17x=17\\x=1`

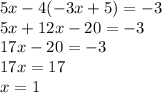
Since we know that the value of x is 1, substitute it into either equation to solve for y:

`y=-3*1+5\\y=-3+5\\y=2\\\\5*1-4y=-3\\5-4y=-3\\-4y=-8\\y=2`

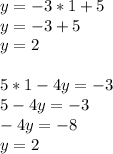
In (x,y) form, the answer is (1,2).

Answers

In short:

1. (1,0)
2. (1,2)