Question

A 0.200-kilogram ball A is traveling at a velocity of 2 meters/second. It hits a 1-kilogram stationary ball B. Which statement is true of the motion of the balls if the collision is elastic? A. The balls separate but move in the same direction. B. The balls stick to each other and move in the initial direction together. C. Ball B moves and ball A stops. D. The balls separate and move in opposite directions.

Answer 1

D

Step-by-step explanation:

Answer 2

As we know that collision is elastic so we will have

`e = 1 = (v_2 - v_1)/(2 - 0)`

`v_2 - v_1 = 2 m/s`

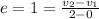
also we can use momentum conservation

`P_(1i) + P_(2i) = P_(1f) + P_(2f)`

`0.200(2m/s) + 0 = 0.200 v_1 + 1v_2`

`0.4 = 0.2v_1 + v_2`

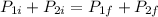
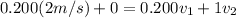
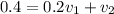
now from above two equations we will have

`1.2 v_1 = -1.6`

`v_1 = -1.33 m/s`

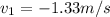
also we have

`v_2 = 0.66 m/s`

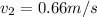
so both balls will separate after collision and move in opposite direction as the final velocities are opposite in sign

So correct answer will be

D. The balls separate and move in opposite directions.