Explanation:
a).
n! is

(n-3)! is
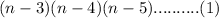
If we divide the two, everything behind (n-3) will just cancel out so we would be left with only
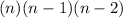
So the answer to a is
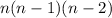
b
Permutations formula of P(6,4) is
6!/(6-4)!, or 6!/2!.

Then we divide by 4!

The answer to b is 15