Question

in a sample of 80 adults, 25 said that they would buy a car from a friend. three adults are selected at random without replacement. find the probability that none of the three would buy a car from a friend.

Answer 1

31.9%

Explanation:

We have that,

Total number of adults = 80

Number of people that would buy a car from a friend = 25

So, the number of people that would not buy a car from a friend = 80-25 = 55.

Since, three people are selected randomly.

Thus, the probability that none of them would buy a car from a friend is given by,

`\frac{\binom{55}{3}}{\binom{80}{3}}`

i.e.
`((55!)/(3!* 52!))/((80!)/(3!* 77!))`

i.e.
`(55!* 3!* 77!)/(3!* 52!* 80!)`

i.e.
`(55* 54* 53)/(80* 79* 78)`

i.e.
`(157410)/(492960)`

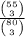
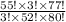
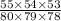
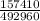
i.e. 0.319

Thus, the probability of people who would not buy a car from a friend is 0.319 i.e. 31.9%