Answer:
csc = 5/4
Explanation:
Assuming that the two given points 3/5 and 4/5 are the two sides of a triangle and a right angle 0 with legs x and y and z as its hypotenuse.
Then using Pythagoras Theorem:
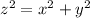
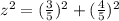
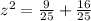
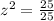

Taking square root on both the sides:
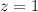
Now,
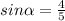
and we know that
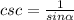
Therefore, csc = 5/4.