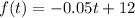Answer:
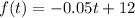
Explanation:
To solve this problem we must pay attention to the following data supplied:
- f It is the number of figurines that remain to be painted.
- t amount of time, in minutes, that Colin spends painting
- Colin takes 20 minutes painting each figurines.
- After painting 60 min, he still has 9 figurines left. f (60) = 9
If he takes 20 minutes painting 1, it means that in 60 minutes he has painted 3 and he has 9 left.
Then, at the beginning he has 9 +3 figurines to be painted.
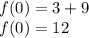
With these two points we can find the function:
If m is the slope of the line, then:
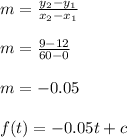
Where by definition
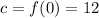
Finally the formula is: