ANSWER
C. 38.83 miles per hour.
EXPLANATION
It was given that, the fuel economy of a car, measured in miles per gallon, is modeled by the function
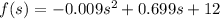
We rewrite the function in the vertex form by completing the square.
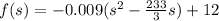

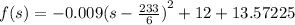
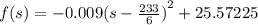
Or
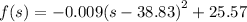
The vertex of this function is
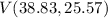
Hence the maximum fuel economy is 25.57 gallons and this occurred at an average speed of 38.83.
The correct answer is C