Question

Let K and T be the current ages of two siblings Katie and Thomas Katie is currently twice the age of Thomas. In 6 years Katie will be 4 times Thomas's current age how old are Katie and Thomas

* Write a system of equations to describe the situation
* find the solution to the system and answer the question

Answer 1

Here, K represents the current age of Katie and T represents the current age of Thomas.

(a)

As per the statement:

Katie is currently twice the age of Thomas.

⇒
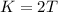`K = 2T` ......[1]

It is also given that in 6 years Katie will be 4 times Thomas's current age.

`K+6 = 4T`

`K+6 = 4T`

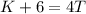
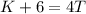
Subtract 6 from both sides we get;

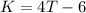`K = 4T-6` ......[2]

A system of equations that describe the situation is:

`K = 2T`

`K+6 = 4T`

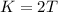
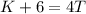
(b)

To find the solution:

equate [1] and [2] we have;

`4T-6 = 2T`

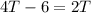
Subtract 2T from both sides we have;

`2T-6 = 0`

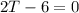
Add 6 to both sides we get;

`2T = 6`

Divide both sides by 2 we have;

T = 3 years

Substitute this in equation [1] we get;

K = 2(3) = 6 years.

Therefore, the current age of Katie and Thomas are 6 years and 3 years.