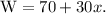Question

Rei is barricading a door to stop a horde of zombies. She stacks boxes of books on a table in front of the door. Each box weighs 30 kilograms, and the table with 8 boxes on top weighs a total of 310 kilograms. The total weight W of the barricade in kilograms is a function of x, the number of boxes Rei stacks on the table. Write the functions formula

Answer 1

Answer: The answer is {W}=70+30x.

Explanation:

{W}=70+30x.

Answer 2

Answer: The answer is
`\textup{W}=70+30x.`

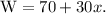
Step-by-step explanation: Given that Rei is barricading a door to stop a horde of zombies by satcking boxes of books on a table in front of the door.

The weight of each box is 30 kilograms and the weight of the table with 8 boxes on the top is 310 kilograms.

So, if 'g' denotes the weight of the table alone, then we have

`\textup{g}+8* 30=310\\\\\Rightarrow \textup{g}+240=310\\\\\Rightarrow \textup{g}=310-240\\\\\Rightarrow \textup{g}=70.`

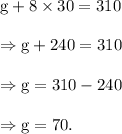
Therefore, the weight of the table without boxes is 70 kilograms.

Now, if 'W' is the total weight of the barricade, then it can be written as a function of 'x', the number of boxes Rei stacks on the table. The expression is as follows -

`\textup{total weight of the barricade}=\textup{weight of the table alone}+30* \textup{number of boxes}.`

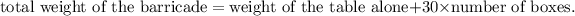
Thus, the required function is

`\textup{W}=70+30x.`