Answer:
15 days
Explanation:
Let x be the number of days needed for B to complete the job. Then x-5 is the number of days needed for A to complete the job.
In 1 day,
- A completes
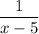 of all work;
of all work; - B completes
 of all work.
of all work.
Hence, in 1 day both A and B complete
 of all work. A and B working together can do a work in 6 days. Then
of all work. A and B working together can do a work in 6 days. Then
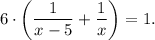
Solve this equation:

If
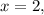 then
then
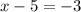 that is impossible. So, B needs 15 days to complete the work alone.
that is impossible. So, B needs 15 days to complete the work alone.