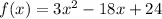Answer:
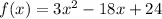
Explanation:
we are given a graph
we can see that graph is of parabola
so, the function will be quadratic in nature
Firstly, we can identify zeros
zeros are x=2 and x=4
now, we can set up function
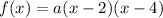
now, we can select anyone point and find 'a'
at x=3 , y=-3
we can use it and find 'a'

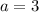
now, we can plug it back
and we get

we can multiply it
and we get