Question

Help!

suppose that all of the points on the circular dartboard shown are equally likely to be hit by a dart. if the radius of the red center circle is 2 in, and the radius of the entire dartboard is 6 in. what is the probability of throwing a dart and hitting the white part of the board?
round your answer to the nearest whole number.

Answer 1

Option (d) i.e. 89%

Explanation:

Area of a circle =
`\pi r^(2)`

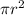
Now, area of inner red circle (radius = 2 in)

=
`\pi(2)^(2) = 4\pi`

And, area of the dartboard (radius = 6 in)

=
`\pi(6)^(2)  = 36\pi`

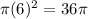
⇒ The area of white part in the dartboard = Area of entire dartboard - inner red circle area = 36π - 4π = 32π

Now, Probability =
`=(No.-of-possible-outcomes)/(Total-outcomes)`

=
`=(32\pi )/(36\pi )`

=
`= (4*8)/(4*9)`

=
`=(8)/(9)`

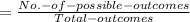
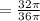
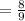
= 88.88% ≈ 89%