Question

If the length, width, and height of a all change by a factor of eight, what happens to the volume of the cube?

Answer 1

The volume will increase by a factor of 512.

Answer 2

It will increase by a factor of 512

Explanation:

The volume of a cube is given by:

`V=w*l*h\\\\Where:\\\\w=Width\\l=Length\\h=Height`

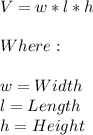
As you can see, the volume of a cube is directly proportional to its width, length and height. In another words if you increase one of them or two of them or all of them, the volume will increase, and if you decrease one of them or two of them or all of them, the volume will decrease. So if you change the length, width, and height by a factor of eight, then:

`V=(8*w)(8*l)(8*h)=8^3wlh=512wlh`

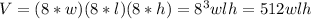
Therefore, as you can see, if you change the length, width, and height by a factor of eight, the original volume of the cube will increase by a factor of 512.