Answer:
B
Explanation:
To find the length of the longest side of the triangle, first sketch the triangle. A graph paper is not needed here.
In this case we have a right-angled triangle, since the ends of the adjacent side has the same y-coordinate of 1 and the opposite side has the same y-coordinate of 5.
In a right-angled triangle, the hypotenuse side is the longest. The length of the hypotenuse side can be found using 2 methods.
1) Pythagoras' Theorem
a² +b²= c²
(adjacent)² +(opposite)²= (hypotenuse)²
Length of adjacent
= 5 -(-2)
= 7 units
Length of opposite side
= 4 -1
= 3 units
(hypotenuse)²
= 7² +3²
= 58
hypotenuse=
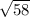
2) Distance formula
Since we know that the hypotenuse side is the longest, we can simply find the length of the hypotenuse side instead of calculating the length of each side.
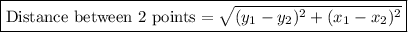
Length of longest side
= distance between (5, 4) and (-2, 1)
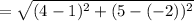

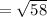
Thus, the length of the longest side is
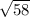 units.
units.