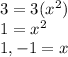Answer:
Depending on what the equation is (see below for options) answers are:
 or -1,1=x
or -1,1=x
Explanation:
Two possibilities are present:
- The base of an exponential function is the number raised to an exponent of x.
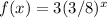 . The base here is 3/8. To find when f(x) = 3, then substitute and solve.
. The base here is 3/8. To find when f(x) = 3, then substitute and solve.
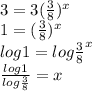
- If this is a quadratic function then it has a base of x.
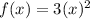 . To find when f(x) = 3 then substitute and solve.
. To find when f(x) = 3 then substitute and solve.