Question

A small publishing company is planning to publish a new book. The production costs will include one-time fixed costs (such as editing) and variable costs (such as printing). There are two production methods it could use. With one method, the one-time fixed costs will total $47,642 , and the variable costs will be $9.25 per book. With the other method, the one-time fixed costs will total $23,792 , and the variable costs will be $20.50 per book. For how many books produced will the costs from the two methods be the same?

Answer 1

2120 books

Explanation:

Let the number of books be
`x`

• For the first method, we can write the cost equation as:

Cost =
`47,642+9.25x`

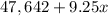
• For the second method, we can write the cost equation as:

Cost =
`23,792+20.50x`

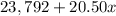
Since we want cost to be same, we equate the 2 equations of cost and solve for x, the number of books, to get our answer:

`47,642+9.25x=23,792+20.50x\\47,642-23,792=20.50x-9.25x\\23,850=11.25x\\x=(23,850)/(11.25)\\x=2120`

The cost for both methods will be same for 2120 books