Question

A man standing on a sidewalk looks up at the roof edge of a building. The building is 40 feet tall and the man stands 12 feet from the building.

What is the measure of the man's angle of inclination from where he stands?

Enter your answer, rounded to the nearest degree, in the box.

_73___

Someone has posted with the answer being 73.3 and someone else put 72.28 but the correct one is 73

Just took the test and in case you need the answer. The answer is 73

Answer 1

Answer: 73 degrees.

Explanation:

1. You can draw a righ triangle as you can see in the figure attached.

2. To calculate man's angle of inclination from where he stands, you must apply the proccedure shown below:

`tan^(-1)(\alpha)=(opposite)/(adjacent)`

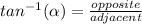
Where:

α is the angle of inclination.

`opposite=40ft\\adjacent=12ft`

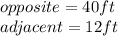
3. When you substitute values, you obtain the following result:

`tan^(-1)(\alpha)=(40)/(12)`

`\alpha=73.30`°

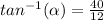
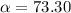4. Rounded to the nearest degree:

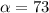`\alpha=73`°