Question

If O N = 8 x − 8 , L M = 7 x + 4 , N M = x − 5 , and O L = 3 y − 6 , find the values of x and y for which LMNO must be a parallelogram. The diagram is not drawn to scale.

Answer 1

x=12 and y=13/3

Explanation:

!!This is the right answer, other one the math is wrong!!

For a parallelogram, opposite sides must be equal.

ON = LM

8x-8 = 7x+4

x = 12

OL = NM

3y-6 = x-5

3y-6 = 12-5

3y = 13

y = 13/3

Answer 2

`x=12\\y=(13)/(3)`

Explanation:

For it to be a parallelogram, opposite sides have to be of same length.

• OL = NM, and
• ON = LM

Using the expressions given, we can make 2 equations:

Equation 1:

`OL=NM\\3y-6=x-5\\-x+3y=1`

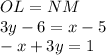
Equation 2:

`ON=LM\\8x-8=7x+4\\`

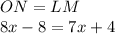
Solving equation 2, we have x:

`8x-8=7x+4\\8x-7x=4+8\\x=12`

Plugging this value of x into equation 1, we can solve for y:

`-x+3y=1\\-(12)+3y=1\\-12+3y=1\\3y=13\\y=(13)/(3)`

So,

`x=12\\y=(13)/(3)`