Question

The perimeter of an equilateral triangle is 77 inches more than the perimeter of a​ square, and the side of the triangle is 55 inches longer than the side of the square. find the side of the triangle.​ (hint: an equilateral triangle has three sides the same​ length.)

Answer 1

143 inches

Explanation:

Let the triangle be
`s` inches long.

Then the perimeter of the equilateral triangle will be
`s+s+s=3s` inches.

Let the length of the square also be
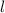`l` inches.

Then the perimeter is
`4l`

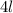
It was given that the perimeter of the equilateral triangle is 77 inches more than the perimeter of the square.

So we can write the relation

`3s=4l+77`

`\Rightarrow 3s-4l=77..eqn1`

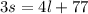
Also, the side of the triangle is 55 inches longer than the side of the square.

So we write
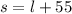`s=l+55` or
`l=s-55...eqn2`

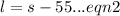
We put equation (2) into equation (1) to get;

`3s-4(s-55)=77`

We expand the bracket to get;

`3s-4s+220=77`

`3s-4s=77-220`

`-s=-143`

`s=143`

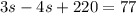
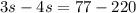
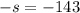
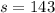
Hence the triangle is 143 inches long.