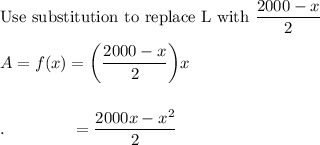Question

Beth has 2000 feet of fencing available to enclose a rectangular field. one side of the field lies along a river, so only three sides require fencing. express the area a of the rectangle as a function of x, where x is the length of the side parallel to the river.

Answer 1

Answer:
`f(x)=(2000x-x^2)/(2)`

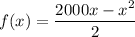
Explanation:

Perimeter (P) is the total length of the fencing which covers 2 sides of one length and 1 side of another length (because the side along the river does not need fencing). So, P = 2L+ x where L is the length and x is the width.

P = 2000 and P = 2L + x → 2000 = 2L + x

`\text{Solve for L:}\\\\2000 = 2L + x\\\\2000-x=2L\\\\(2000-x)/(2)=L`

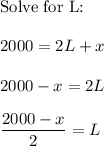
Area (A) is length x width → A = L × x

`\text{Use substitution to replace L with}\ (2000-x)/(2)\\\\A = f(x)=\bigg((2000-x)/(2)\bigg)x\\\\\\.\qquad \qquad =(2000x-x^2)/(2)`