Question

Match each set of vertices with the type of triangle they form.

Tiles

A(2, 0), B(3, 2), C(5, 1)

obtuse scalene triangle

A(4, 2), B(6, 2), C(5, 3.73)

isosceles right triangle

A(-5, 2), B(-4, 4), C(-2, 2)

right triangle

A(-3, 1), B(-3, 4), C(-1, 1)

acute scalene triangle

A(-4, 2), B(-2, 4), C(-1, 4)




i need help plz and it should be correct for all these points

Answer 1

Explanation:

Given the following pair of vertices we have to find the nature

first pair of vertices of triangle A(2, 0), B(3, 2), C(5, 1)

By distance formula

`AB=√((3-2)^2+(2-0)^2)=\sqrt5`

`BC=√((5-3)^2+(1-2)^2)=\sqrt5`

`AC=√((5-2)^2+(1-0)^2)=\sqrt10`

gives
`AC^2=AB^2+BC^2`

Hence, right angled triangle

A(4, 2), B(6, 2), C(5, 3.73)

`AB=√((6-4)^2+(2-2)^2)=2`

`BC=√((5-6)^2+(3.73-2)^2)=\sqrt3.993`

`AC=√((5-4)^2+(3.73-2)^2)=\sqrt3.992`

Isosceles triangle

A(-5, 2), B(-4, 4), C(-2, 2)

`AB=√((-4+5)^2+(4-2)^2)=\sqrt5`

`BC=√((-2+4)^2+(2-4)^2)=\sqrt8`

`AC=√((5-2)^2+(1-0)^2)=3`

Scalene triangle

A(-3, 1), B(-3, 4), C(-1, 1)

`AB=√((-3+3)^2+(4-1)^2)=3`

`BC=√((-1+3)^2+(1-1)^2)=2`

`AC=√((-1+3)^2+(1-1)^2)=2`

Isosceles triangle

A(-4, 2), B(-2, 4), C(-1, 4)

`AB=√((-2+4)^2+(4-2)^2)=\sqrt8`

`BC=√((-1+2)^2+(4-4)^2)=1`

`AC=√((-1+4)^2+(4-2)^2)=\sqrt13`

Scalene triangle

Answer 2

Answer: The calculations are done below.

Step-by-step explanation:

(i) Let the vertices be A(2,0), B(3,2) and C(5,1). Then,

`AB=√((2-3)^2+(0-2)^2)=√(5),\\\\BC=√((3-5)^2+(2-1)^2)=√(5),\\\\CA=√((5-2)^2+(1-0)^2)=√(10).`

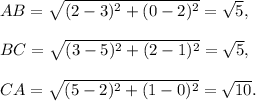
Since, AB = BC and AB² + BC² = CA², so triangle ABC here will be an isosceles right-angled triangle.

(ii) Let the vertices be A(4,2), B(6,2) and C(5,3.73). Then,

`AB=√((4-6)^2+(2-2)^2)=√(4)=2,\\\\BC=√((6-5)^2+(2-3.73)^2)=√(14.3729),\\\\CA=√((5-4)^2+(3.73-2)^2)=√(14.3729).`

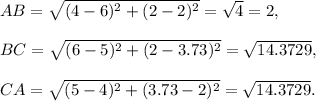
Since, BC = CA, so the triangle ABC will be an isosceles triangle.

(iii) Let the vertices be A(-5,2), B(-4,4) and C(-2,2). Then,

`AB=√((-5+4)^2+(2-4)^2)=√(5),\\\\BC=√((-4+2)^2+(4-2)^2)=√(8),\\\\CA=√((-2+5)^2+(2-2)^2)=√(9).`

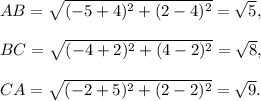
Since, AB ≠ BC ≠ CA, so this will be an acute scalene triangle, because all the angles are acute.

(iv) Let the vertices be A(-3,1), B(-3,4) and C(-1,1). Then,

`AB=√((-3+3)^2+(1-4)^2)=√(9)=3,\\\\BC=√((-3+1)^2+(4-1)^2)=√(13),\\\\CA=√((-1+3)^2+(1-1)^2)=\sqrt 4.`

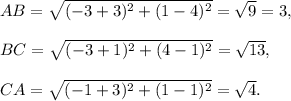
Since AB² + CA² = BC², so this will be a right angled triangle.

(v) Let the vertices be A(-4,2), B(-2,4) and C(-1,4). Then,

`AB=√((-4+2)^2+(2-4)^2)=√(8),\\\\BC=√((-2+1)^2+(4-4)^2)=√(1)=1,\\\\CA=√((-1+4)^2+(4-2)^2)=√(13).`

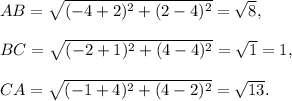
Since AB ≠ BC ≠ CA, and so this will be an obtuse scalene triangle, because one angle that is opposite to CA will be obtuse.

Thus, the match is done.