Question

The graph shows the distance cars A and B travel.

Which statement is true about the unit rates shown in the graph?
(a) The unit rate for car B is greater because the slope is greater than the slope for car A
(b) The unit rate for car A is greater because the slope is less than the slope for car B
(c) The unit rate for car A is greater because the slope is greater than the slope for car B
(d) The unit rate for car B is greater because the slope is less than the slope for car A
PLEASE HELP

Answer 1

That will be A bc B is much longer than A

Answer 2

(a) The unit rate for car B is greater because the slope is greater than the slope for car A.

Explanation:

We have been given a graph that represents the distance cars A and B travel. We are asked to choose the correct option about our given graphs.

Since slope represents the change in y-value with respect to change in per unit x value, so slope is also unit rate of the graph.

Let us find the slope of car A using points (8,5) and (0,0).

`\text{Slope}=(y_2-y_1)/(x_2-x_1)`

`\text{Slope of Car A}=(5-0)/(8-0)`

`\text{Slope of Car A}=(5)/(8)`

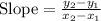
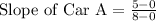
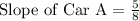
Let us find the slope of car B using points (5,8) and (0,0).

`\text{Slope of Car B}=(8-0)/(5-0)`

`\text{Slope of Car B}=(8)/(5)`

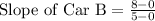
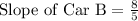
We can see that slope of car B is greater than slope of car A, therefore, option A is the correct choice.