Question

Solve the compound inequality.

3x+7≥ and 8x-3≤21

Any help is appreciated!

Answer 1

The solution of the compound inequality is the interval
`[-3,3]`

Explanation:

we have

`3x+7\geq -2` -----> inequality A

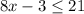`8x-3\leq 21` ----> inequality B

Step 1

Solve the inequality A

`3x\geq -2-7`

`x\geq -9/3`

`x\geq -3`

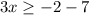
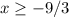
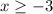
The solution of the inequality A is the interval---------> [-3,∞)

All real numbers greater than or equal to
`-3`

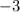
Step 2

Solve the inequality B

`8x\leq 21+3`

`x\leq 24/8`

`x\leq 3`

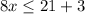
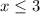
The solution of the inequality B is the interval---------> (-∞,3]

All real numbers less than or equal to
`3`

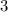
Step 3

Find the solution of the compound inequality

[-3,∞) ∩ (-∞,3]=[-3,3]