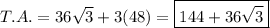Question

What is the total area of this figure
( _ _ _ + _ _ sqrt _)

Answer 1

Look at the picture.

We have in a base an equilateral triangle.

The formula of the area of an equilateral triangle:

`A_B=(a^2\sqrt3)/(4)`

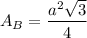
We have a = 12. Substitute:

`A_B=(12^2\sqrt3)/(4)=(144\sqrt3)/(4)=36\sqrt3`

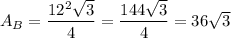
The lateral side is a isosceles triangle. The formula of the area of a triangle is:

`A_\triangle=(1)/(2)bh`

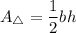
We must calculate the length of h using the Pythagorean theorem:

`h^2+6^2=10^2`

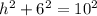
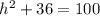`h^2+36=100` subtract 36 from both sides

`h^2=64\to h=√(64)\to h=8`

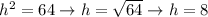
We have b = 13 and h = 8. Substitute:

`A_\triangle=(1)/(2)(12)(8)=(6)(8)=48`

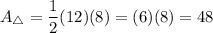
The Total Area:

`T.A.=A_B+3A_\triangle`

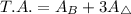
Substitute:

`T.A.=36\sqrt3+3(48)=\boxed{144+36\sqrt3}`