Question

Jack looks at a clock tower from a dictance and determines the the angle of elevation of the top of the tower is 40°. John who is standing 20 melters from jack as shown in the diagram, determines that the angle of elevation to the top of the tower is 60°. If jacks and johns eyes are 1.5 meters from the ground and the distance from jacks eyes to the top of the tower is 50.64 meters, how far is johns from the base of the tower?

Answer 1

D) 18.8

Explanation:

i got it right on edmentum and plato both the same

Answer 2

As per the given statement:

The distance from jack eyes to the top of the tower = 50.64 m

Also, jack and john eyes are 1.5 m from the ground.

Labelled diagram as shown in the attachment

Let BC = x m be the distance of the jack from the base of the tower and

AG = y m be the distance above 1.5 m from the the ground.

Using tangent ratio:

`\tan \theta = \frac{\text{Opposite side}}{\text{Adjacent side}}`

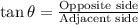
In triangle AGE;

`\tan 40^(\circ) = (AG)/(EG)`

Here, AG = y m and EG = 20+x m

then;

`\tan 40^(\circ) = (y)/(20+x)`

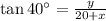
or we can write this as;

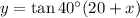`y = \tan 40^(\circ) (20+x)` ........[1]

Similarly, in AGF;

`\tan 60^(\circ) = (AG)/(FG)`

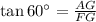
then;

`\tan 60^(\circ) = (y)/(x)`

`√(3) = (y)/(x)`

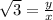
or

`y = √(3)x` ......[2]

Substitute equation [2] into the equation [1], to solve for x;

`√(3)x= \tan 40^(\circ)(20+x)`

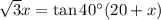
or

`√(3)x= 0.84(20+x)`

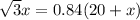
Using distributive property:
`a\cdot (b+c) = a\cdot b+ a\cdot c`

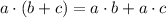
we have;

`1.732x = 16.8 + 0.84x`

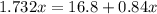
Subtract 0.84 x from both sides we get;

`0.892x = 16.8`

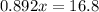
Divide both sides by 0.892 we get;

`x \approx 18.8 m`

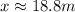
Therefore, 18.8 meter johns from the base of the tower.