Answer:
Linear function :
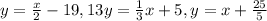
Non - Linear function :

Explanation:
Linear function : If a function has either one or two variables with degree 1, then it is known as linear function. The graph of a linear function is always a straight line.
Non - Linear function : If a function has either one or two variables with exponents, then it is known as non-linear function. The graph of a non-linear function is a curve.
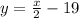
Here, degree of both variables is 1. So, it is a linear function.
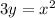
Here, degree of y is 1 and degree of x is 2. So, it is a non-linear function.

Here, degree of both variables is 1. So, it is a linear function.
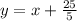
Here, degree of both variables is 1. So, it is a linear function.

Here, degree of y is 3 and degree of x is 1. So, it is a non-linear function.