Answer:
1)
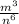
2)
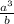
3)
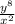
Explanation:
To evaluate or simplify expressions with exponents, we use exponent rules.
1. An exponent is only a short cut for multiplication. It simplifies how we write the expression.
2. When we multiply terms with the same bases, we add exponents.
3. When we divide terms with the same bases, we subtract exponents.
4. When we have a base to the exponent of 0, it is 1.
5. A negative exponent creates a fraction.
6. When we raise an exponent to an exponent, we multiply exponents.
7. When we have exponents with parenthesis, we apply it to everything in the parenthesis.
We will use these rules to simplify.
Problems:
1)
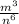
n^-6 has a negative exponent and should be moved to the denominator while p^0 is equal to 1
2)
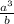
Divide each base by subtracting exponents. a^4-1 = a^3 and b^-3--2=b^-1. B has a negative exponent so move it into the denominator.
3)
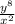
Simplify inside the parenthesis first by adding exponents of same bases x^-2+3 = x. Then apply the outside exponent using power to a power rule and negative exponent rule.