Answer: Horizontal Speed = 4.6 m/s
Vertical Speed = 1.7 m/s
Step-by-step explanation:
Jumper's velocity = 5 m/s
Angle = 20 degrees
the angle is theta, so theta = 20 degrees
Theta is the angle between the hypothenuse and the adjacent side.
The horizontal side will be the adjacent side, and the vertical side will be the opposite side.
The hypothenuse = 5 m/s
First, let's calculate the adjacent side (horizontal speed)
We have cosine of theta, which is equal to adjacent side / hypothenuse
So,
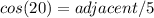
Multiply 5 on both sides, to get the 5 on the right side canceled.
5cos(20) = adjacent side.
4.6 = adjacent side
Horizontal Speed = 4.6 m/s
Now, let's calculate the vertical speed.
To do that, we need to calculate the opposite side.
We can calculate the opposite side with the help of sin.
The sin of theta equals to opposite side / hypothenuse
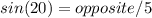
We do the same thing, multiply 5 on both sides.
5sin(20) = opposite
1.7 = opposite
Vertical Speed = 1.7 m/s
Hope I Helped!