Answer: The new pressure of the gas sample once the gas temperature in the jar 0.9424 atm.
Step-by-step explanation:
Volume of the gas = 250 mL
Pressure of the gas at
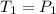
Pressure of the gas at
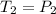
At the constant volume pressure of the gas is directly proportional to the temperature of the gas in Kelvins that is Gay-Lussac's Law
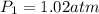 ,
,

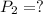 ,
,
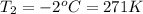
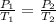
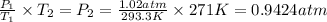
The new pressure of the gas sample once the gas temperature in the jar 0.9424 atm.