Question

Determine the intercepts of the line that passes through the following points (10,20), (15,12), to (20,4).

Answer 1

The y -intercept is (0,36).

The x-intercept is (22.5,0)

Explanation:

To find the intercepts, write the equation in slope intercept form. First, find the slope of the line using the two points. Find the difference in y values over the difference in x values.

`(y_2-y_1)/(x_2-x_1) = (4-12)/(20-15)=(-8)/(5)`

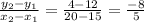
Write the equation using the slope m=-8/5 and the point slope form.

`y-y_1=m(x-x_1)\\y-20=(-8)/(5)(x-10)`

This is the equation of the line. You can convert it to slope intercept form:

`y-20=(-8)/(5)(x-10)\\y-20 = (-8)/(5)x + 16\\y=(-8)/(5)x +36`

The y -intercept is (0,36).

To find the x-intercept, plug in 0 for y.

0=-8/5 x +36

-36 = -8/5x

-36 * -5/8 = x

22.5 = x

The x-intercept is (22.5,0)