Answer:
see explanation
Explanation:
the exterior angle = 180° - interior angle
(17)
exterior angle = 180° - 144° = 36°
The sum of the exterior angles of a polygon = 360°
Hence the number of sides n is
n =
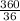 = 10
= 10
(18)
exterior angle = 180° - 156° = 24°
n =
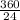 = 15
= 15
(19)
exterior angle = 180° - 160° = 20°
n =
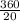 = 18
= 18
In the following 2 questions we are using that the sum of the interior angles of a quadrilateral is 360°
(20)
summing the angles and equating to 360
2x + 15 + 3x - 20 + x + x + 15 = 360
7x + 10 = 360 ( subtract 10 from both sides )
7x = 350 ( divide both sides by 7 )
x = 50
∠J = 2x + 15 = 100 + 15 = 115°
∠K = 3x - 20 = 150 - 20 = 130°
∠M = x = 50°
∠N = x + 15 = 50 + 15 = 65°
(21)
6x - 4 + 2x + 8 + 6x - 4 + 2x + 8 = 360
16x + 8 = 360 ( subtract 8 from both sides )
16x = 352 ( divide both sides by 16 )
x = 22
∠R = ∠T = 6x - 4 = 132 - 4 = 128°
∠S = ∠U = 2x + 8 = 44 + 8 = 52°