Answer:
The point slope equation be
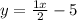 .
.
Explanation:
The point slope equation of the line is given by .
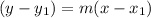
Where m is the slope .
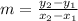
![m =\frac{1-(-3){12-4}]()

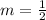
As given
The point-slope form of the equation of the line that passes through (4, -3) and (12, 1) is
 .
.
Putting the values in the point slope equation .

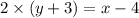
2y + 6 = x - 4
2y = x - 4 - 6
2y = x -10
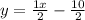
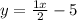
Therefore the point slope equation be
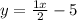 .
.